

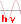





The work of Louis de Broglie, Erwin Schrödinger and Werner Heisenberg led to mathematical calculations resulting in a famous equation whose solutions are all electron energy states that can be occupied (all energies electrons may have ..) about any nucleus. As in the case of Bohr's theory, energy statements provided by these calculations are numerable:
The allowed energy states for electrons are designated by several integers:
.The principal quantum number $n$ $ = $ $1,2,3,4...$ designates the shells (levels).
So each atom has a first layer $n$ $=$ $1$ (from the nucleus), a second $n$ $=$ $2$ etc ... Traditionally, the layers can still be identified by the following capital letters:
| n | name |
| 1 | K |
| 2 | L |
| 3 | M |
| 4 | N |
| 5 | O |
The secondary (azimuthal, angular) quantum number $l = n-1,n-2...0$ designates the subshells (sublevels).
On the first shell $n$ $=$ $1$, there is therefore an subshell $l$ $=$ $0$, on the second $n=2$ two subshells, namely $l$ $=$ $1$ and $l$ $=$ $0$, on the third $n=3$ three subshells, namely the $l$ $=$ $2$, $l$ $=$ $1$ and $l$ $=$ $0$ etc. .. Traditionally, sub-layers can still be identified by the following lowercase letters:
| l | name |
| 0 | s |
| 1 | p |
| 2 | d |
| 3 | f |
The three subshells of the $M$ shell ($n=3$) are thus written: $3s,\; 3p,\; 3d$
The magnetic quantum number $m$ $=$ $-l,-l+1,...,0,...,l-1,l$ designates the obitals (orbitals).
A subshell of type $f$ $(l=3)$ (in any shell) has for example always seven numbered obitals $m=-3$ $m=-2$ $m=-1$ $m=0$ $m=1$ $m=2$ $m=3$ A subshell of type $d$ $(l=2)$ (in any shell) has for example always five numbered obitals $m=-2$ $m=-1$ $m=0$ $m=1$ $m=2$ A subshell of type $p$ $(l=1)$ (in any shell) has for example always three numbered obitals $m=0$, $m=1$. A subshell of type $s$ $(l=0)$ (in any shell) has for example always one numbered obital $m=0$ Traditionally, the only obital of an subshell $s$ is still designated by the symbol $s$ The three obitals of a subshell $p$ are designated by $p_x$ $p_y$ $p_z$ The obitals of subshells $d$ et $f$ have more complicated designations.
The spin quantum number $s$ $= $ $\frac{1}{2},-\frac{1}{2}$ designates the spin states.
Therefore, in each obital we find two spin states .
In the second shell $L$ $(n$ $=$ $2)$, we find: One subshell $s$ $(l$ $=$ $0)$ and one subshell $p$ $(l$ $=$ $1)$ The $s$ subshell contains one obital $s$ $(m$ $=$ $0)$ with two spin states. The $p$ subshell contains three obitals $p_x$ $(m=-1)$ $p_y$ $(m=0)$ $p_z$ $(m=1)$ each with two spin states. In total, the second layer has 8 spin states.